Table of Contents
Algebra
- Expressions and Formulae
- Writing Formulae
- Solving Formulae
- Changing the Subject of a Formulae
- Inequalities
- Number Lines
- Solving Inequalities
- Equations
- Solving Equations
- Equations with Brackets
- Equations with Fraction
- Graphs
- Straight Line Graphs ( y = mx + c )
- Patterns and Sequences
- Basic Patterns
- Linear Sequences & nth term
- Quadratic Equations
Writing Formula
L.O to be able to write and understand algebraic formulae for word problems Formulae are a way of rewriting problems or describing a rule. They are written in algebraic form which means that letters are used. However the main element that shows that something is a formulae is that it will contain an = sign. Without even thinking about it, you have already been using formulae in maths before; for instance when working out the area of a triangle. 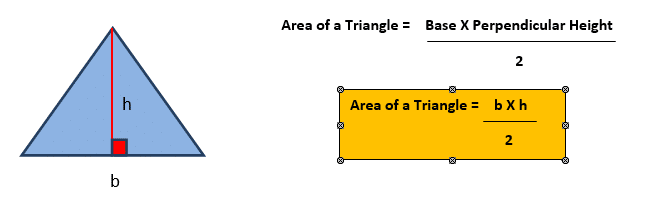 Here the base and perpendicular heighthave been abbreviated, shortened, to b and h. This is exactly the point of formulas, to make solving problems later on easier, in such a way that any measurement can be applied to the formulae. Similarly you have already used formulas when working out other problems (notably areas) for example:
Here the base and perpendicular heighthave been abbreviated, shortened, to b and h. This is exactly the point of formulas, to make solving problems later on easier, in such a way that any measurement can be applied to the formulae. Similarly you have already used formulas when working out other problems (notably areas) for example: 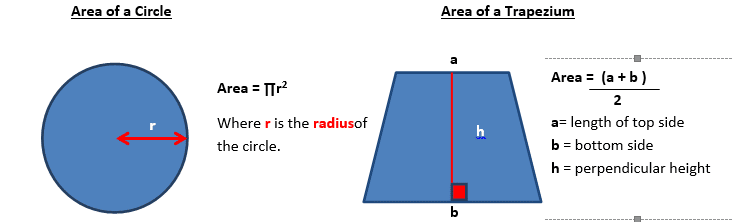 Note how each of these formulas have an = sign. Now you are familiar with what formulas look like, it is now time to substitute values into formula As shown above, formulae can be used to help solve problems. However you need to be able to write formulae before you can solve or manipulate them further. For instance if in a supermarket you see an apple for 20p and you wanted to buy 3apples, you would mentally apply the following calculation: 1apple is 20p 2 apples are (20 X 2) = 40p 3 apples are (20 X 3) = 60p So the formula to work out the cost of buying a number of apples is: .
Note how each of these formulas have an = sign. Now you are familiar with what formulas look like, it is now time to substitute values into formula As shown above, formulae can be used to help solve problems. However you need to be able to write formulae before you can solve or manipulate them further. For instance if in a supermarket you see an apple for 20p and you wanted to buy 3apples, you would mentally apply the following calculation: 1apple is 20p 2 apples are (20 X 2) = 40p 3 apples are (20 X 3) = 60p So the formula to work out the cost of buying a number of apples is: .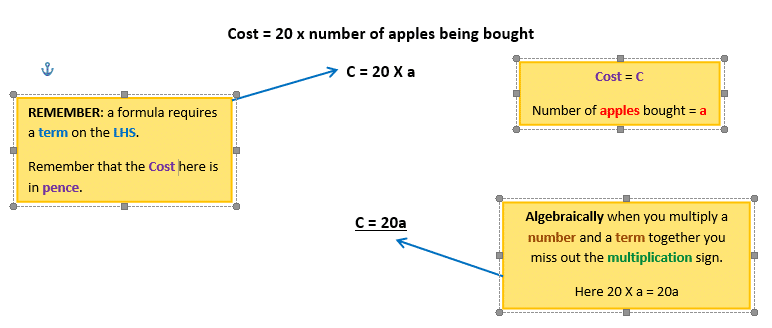 Cake baskets are £50 each. Samantha has many friends coming and wants a formula to help calculate the cost. In this example you would do as before: 1 basket = £50 2 baskets are (50 X 2) = £100 3 baskets are (50 X 3) = £150
Cake baskets are £50 each. Samantha has many friends coming and wants a formula to help calculate the cost. In this example you would do as before: 1 basket = £50 2 baskets are (50 X 2) = £100 3 baskets are (50 X 3) = £150
Cost = 50 x number of basket being bought
 Example 1: Jane is going to order some parcels online. Each parcel costs £5 but also has a small administration fee of £2.50 for each order processed. Write a formula to calculate the cost of one of Jane’s orders.
Example 1: Jane is going to order some parcels online. Each parcel costs £5 but also has a small administration fee of £2.50 for each order processed. Write a formula to calculate the cost of one of Jane’s orders.  Example 2: Aqif is having a banquet. The cost of a 3 course meal per adults is £15, per child is £6 and for younger children is £3. There is also a fee of £35 for hiring the hall. Write a formula to calculate the cost of Aqif’s banquet.
Example 2: Aqif is having a banquet. The cost of a 3 course meal per adults is £15, per child is £6 and for younger children is £3. There is also a fee of £35 for hiring the hall. Write a formula to calculate the cost of Aqif’s banquet. 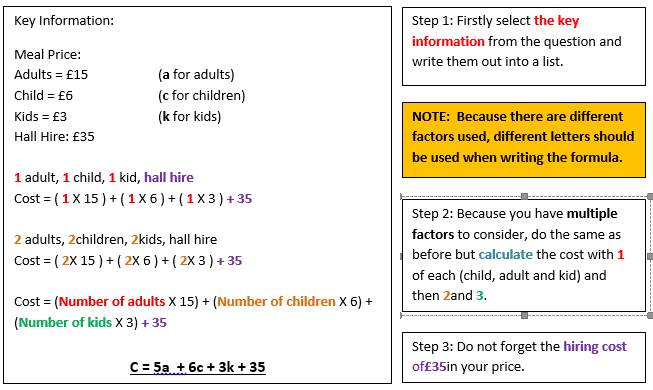 Example 3: A group of 6 friends are making a fruit basket containing: apples at 60p each, pears at 40p and oranges at 80p each. They decide to split the cost of the basket equally between them. Write a formula for the cost of each person.
Example 3: A group of 6 friends are making a fruit basket containing: apples at 60p each, pears at 40p and oranges at 80p each. They decide to split the cost of the basket equally between them. Write a formula for the cost of each person. 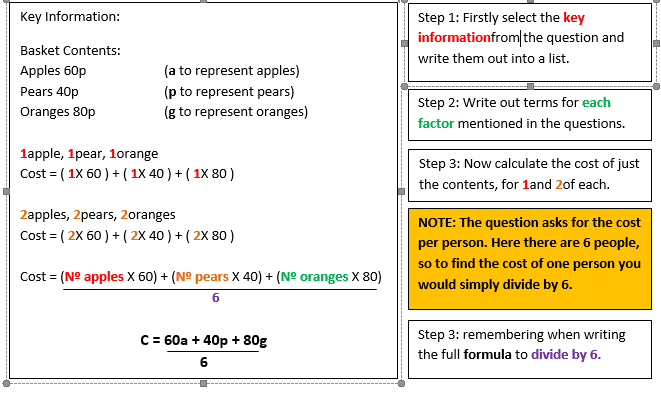 Example 4: Sarah is having a birthday party and wants to make 5 bags that contain a different number of toys (30p), sweets (50p) and messages (55p). Write a formula for a given number of items for 5 party bags.
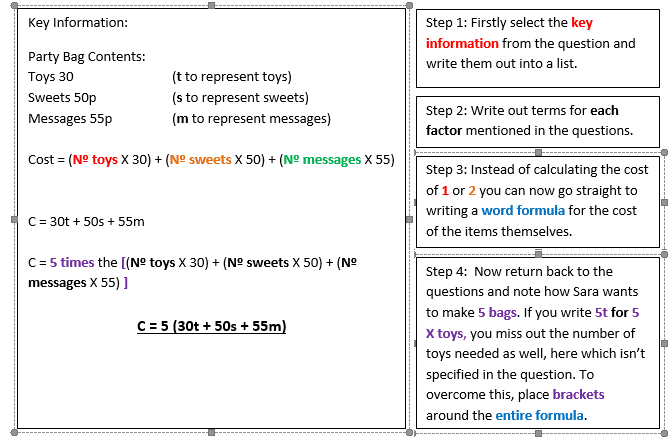
Example 4: Sarah is having a birthday party and wants to make 5 bags that contain a different number of toys (30p), sweets (50p) and messages (55p). Write a formula for a given number of items for 5 party bags.